The time value of money is a financial concept that holds that the value of a dollar today is worth more than the value of a dollar in the future. This is true because money you have now can be invested for a financial return, also the impact of inflation will reduce the future value of the same amount of money.
The time value of money is a fundamental concept in finance that refers to the greater value of money in the present than the same sum in the future This is due to the potential to invest money and earn interest over time The time value of money affects everyday personal and business decisions from calculating loan payments to retirement planning. Let’s explore this important principle with some practical examples.
What is Time Value of Money?
The time value of money is based on the premise that money available now is worth more than the same amount in the future because of its potential earning capacity. For example $100 today can be invested at 5% annual interest and grow to $105 in one year. By accepting $100 now rather than $105 in one year you give up the opportunity to earn 5% interest. This is called the present discounted value.
Some key tenets of time value of money:
-
A dollar today is worth more than a dollar tomorrow because today’s dollar can be invested to start earning interest immediately.
-
Money loses purchasing power over time due to inflation. A dollar today buys more than it will in the future.
-
The longer the investment time period, the greater the opportunity for compounding interest.
Understanding this concept allows individuals and businesses to evaluate financial opportunities like loans, investment returns and savings.
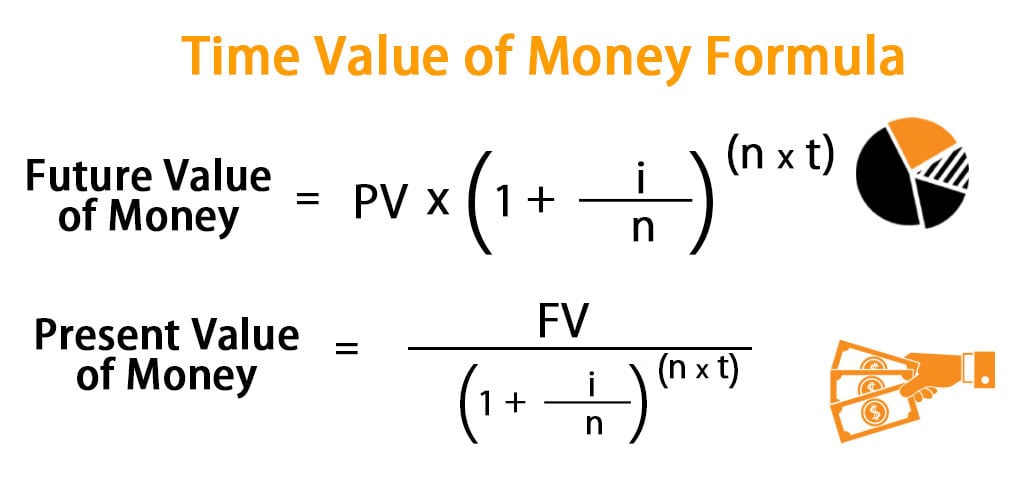
Time Value of Money Formula
The time value of money formula considers four variables:
- Present Value (PV) – The current value of money
- Future Value (FV) – The value of money at a future date
- Interest Rate (i) – The interest rate for the investment
- Number of Periods (n) – The number of periods money is invested
The formula shows how an amount today grows to some future value with compound interest:
FV = PV (1 + i)n
Where:
PV = Present Value
i = Periodic Interest Rate
n = Number of Periods
FV = Future Value
For example, $1,000 invested today at 6% annual interest for 5 years would grow to:
FV = $1,000 (1 + 0.06)5 = $1,338
This basic formula can be manipulated to determine any of the variables when the other values are known.
Why is Time Value of Money Important?
Understanding time value of money allows for better financial decision making in areas like:
-
Savings – Money should be invested, not spent, to maximize growth.
-
Borrowing – Paying off debts early minimizes interest expenses.
-
Budgeting – Future costs will be higher due to inflation.
-
Investments – Compounding interest boosts returns over long periods.
-
Retirement – Starting savings early means more compounding.
-
Salaries – Pay raises should exceed inflation to boost real income.
-
Purchasing – Paying cash costs less than borrowing with interest.
Time Value of Money Examples
Here are some practical examples that demonstrate the time value of money concept:
Example 1: Individual Loan Decision
John can take out a $10,000 one-year loan at 10% interest, with the full balance and interest due at maturity. What is the loan’s future value?
- PV = $10,000
- i = 10%
- n = 1 year
- FV = $10,000 (1 + 0.10)1 = $11,000
The $10,000 loan will cost John $11,000 in one year. The $1,000 difference reflects the time value of money – the interest expense for borrowing money now and repaying it later.
John can use this calculation to evaluate whether the loan is affordable given his financial situation. The high 10% interest rate also indicates John should shop around to find a lower rate if possible.
Example 2: Mortgage Payoff Decision
Carol has a $200,000 mortgage at 4% annual interest with 20 years remaining. She recently inherited $50,000 and is debating whether to:
- Invest the $50,000 at 6% return
- Make a lump sum payment on her mortgage
If she invests the money, after 20 years it would grow to:
- PV = $50,000
- i = 6%
- n = 20 years
- FV = $50,000 (1 + 0.06)20 = $134,100
If she puts the $50,000 toward her mortgage, she will save:
- Mortgage balance = $200,000
- Interest rate = 4%
- Interest savings per year = $50,000 x 0.04 = $2,000
- Interest savings over 20 years = $2,000 x 20 = $40,000
In this case, Carol is better off investing the $50,000 as it will earn more than the interest she would save on her mortgage by paying it down faster. This demonstrates the importance of considering the time value of returns on different uses of money.
Example 3: Retirement Planning
Rachel, 25, wants to retire at 65 and expects to need $3 million saved. She plans to invest $5,000 per year indexed at 3% for inflation, earning 6% annual returns. How much will she have at age 65?
- Annual investment = $5,000
- Interest rate = 6%
- Number of years = 65 – 25 = 40
- Inflation rate = 3%
With 3% inflation, Rachel’s contributions will increase by 3% per year. Using a formula that accounts for changing periodic payments, her future value at 65 is:
FV = $5,000 (1.06^40 – 1) / (0.06 – 0.03) = $3,130,713
By understanding compound returns, Rachel realizes she needs to invest more than $5,000 annually to reach her goal. Getting an early start allows compounding to work in her favor.
Example 4: Comparing Job Offers
James is comparing two job offers with the same annual starting salary of $50,000:
-
Job A – $50,000 per year with 3% annual raises
-
Job B – $50,000 per year with no raises but a $5,000 year-end bonus
-
Expected tenure at job = 5 years
Accounting for the time value of money with a 3% discount rate, the job offers compare as:
- Job A = $50,000 (PV of annuity, 3% raises for 5 years) = $257,400
- Job B = 5 annual bonuses discounted to today’s dollars at 3% = $22,300 + $50,000 x 5 years discounted at 3% = $221,200
Despite the same starting salary, Job A is worth over $36,000 more than Job B after factoring in the time value of guaranteed raises compared to a discretionary bonus.
Key Considerations
While the time value of money is essential for informed financial decision making, some important factors to keep in mind include:
-
Estimating future returns is difficult and actual results may differ significantly. Use reasonable conservative estimates as inputs.
-
Inflation reduces purchasing power over time. Factor in inflation to avoid understating future costs or overvaluing future returns.
-
The timing of cash flows matters. Earlier is better for income received, later is better for expenses paid.
-
Tax implications may affect the choice between investment returns versus interest savings in some scenarios. Consult a tax pro.
Calculating Future Value
The above calculation, then, is equivalent to the following equation:
Future Value = $ 1 0 , 0 0 0 × ( 1 + 0 . 0 4 5 ) × ( 1 + 0 . 0 4 5 ) begin{aligned} &text{Future Value} = $10,000 times ( 1 + 0.045 ) times ( 1 + 0.045 ) \ end{aligned} Future Value=$10,000×(1+0.045)×(1+0.045)
Think back to math class and the rule of exponents, which states that the multiplication of like terms is equivalent to adding their exponents. In the above equation, the two like terms are (1+ 0.045), and the exponent on each is equal to 1. Therefore, the equation can be represented as the following:
Future Value = $ 1 0 , 0 0 0 × ( 1 + 0 . 0 4 5 ) 2 begin{aligned} &text{Future Value} = $10,000 times ( 1 + 0.045 )^2 \ end{aligned} Future Value=$10,000×(1+0.045)2
We can see that the exponent is equal to the number of years for which the money is earning interest in an investment. So, the equation for calculating the three-year future value of the investment would look like this:
Future Value = $ 1 0 , 0 0 0 × ( 1 + 0 . 0 4 5 ) 3 begin{aligned} &text{Future Value} = $10,000 times ( 1 + 0.045 )^3 \ end{aligned} Future Value=$10,000×(1+0.045)3
However, we dont need to keep on calculating the future value after the first year, then the second year, then the third year, and so on. You can figure it all at once, so to speak. If you know the present amount of money you have in an investment, its rate of return, and how many years you would like to hold that investment, you can calculate the future value (FV) of that amount. Its done with the equation:
FV = PV × ( 1 + i ) n where: FV = Future value PV = Present value (original amount of money) i = Interest rate per period n = Number of periods begin{aligned} &text{FV} = text{PV} times ( 1 + i )^ n \ &textbf{where:} \ &text{FV} = text{Future value} \ &text{PV} = text{Present value (original amount of money)} \ &i = text{Interest rate per period} \ &n = text{Number of periods} \ end{aligned} FV=PV×(1+i)nwhere:FV=Future valuePV=Present value (original amount of money)i=Interest rate per periodn=Number of periods
Future Value Basics
If you choose Option A and invest the total amount at a simple annual rate of 4.5%, the future value of your investment at the end of the first year is $10,450. We arrive at this sum by multiplying the principal amount of $10,000 by the interest rate of 4.5% and then adding the interest gained to the principal amount:
$ 1 0 , 0 0 0 × 0 . 0 4 5 = $ 4 5 0 begin{aligned} &$10,000 times 0.045 = $450 \ end{aligned} $10,000×0.045=$450
$ 4 5 0 + $ 1 0 , 0 0 0 = $ 1 0 , 4 5 0 begin{aligned} &$450 + $10,000 = $10,450 \ end{aligned} $450+$10,000=$10,450
You can also calculate the total amount of a one-year investment with a simple manipulation of the above equation:
OE = ( $ 1 0 , 0 0 0 × 0 . 0 4 5 ) + $ 1 0 , 0 0 0 = $ 1 0 , 4 5 0 where: OE = Original equation begin{aligned} &text{OE} = ( $10,000 times 0.045 ) + $10,000 = $10,450 \ &textbf{where:} \ &text{OE} = text{Original equation} \ end{aligned} OE=($10,000×0.045)+$10,000=$10,450where:OE=Original equation
Manipulation = $ 1 0 , 0 0 0 × [ ( 1 × 0 . 0 4 5 ) + 1 ] = $ 1 0 , 4 5 0 begin{aligned} &text{Manipulation} = $10,000 times [ ( 1 times 0.045 ) + 1 ] = $10,450 \ end{aligned} Manipulation=$10,000×[(1×0.045)+1]=$10,450
Final Equation = $ 1 0 , 0 0 0 × ( 0 . 0 4 5 + 1 ) = $ 1 0 , 4 5 0 begin{aligned} &text{Final Equation} = $10,000 times ( 0.045 + 1 ) = $10,450 \ end{aligned} Final Equation=$10,000×(0.045+1)=$10,450
The manipulated equation above is simply a removal of the like-variable $10,000 (the principal amount) by dividing the entire original equation by $10,000.
If the $10,450 left in your investment account at the end of the first year is left untouched and you invested it at 4.5% for another year, how much would you have? To calculate this, you would take the $10,450 and multiply it again by 1.045 (0.045 +1). At the end of two years, you would have $10,920.25.
Time Value of Money – Present Value vs Future Value
What is the time value of money?
The time value of money is an important concept to keep in mind because your money, once invested, can grow over time. Even if you were to just put it into a CD or savings account, the money can earn compound interest, and the impact of compounding on investment growth can be significant.
How do you calculate time value of money?
You can use the following formula to calculate the time value of money: FV = PV x [1 + (i / n)] (n x t). The future value of money isn’t the same as present-day dollars. And the same is true about money from the past. This phenomenon is known as the time value of money.
Does the time value of money influence the decision-making process?
It would be hard to find a single area of finance where the time value of money does not influence the decision-making process. The time value of money is the central concept in discounted cash flow (DCF) analysis, which is one of the most popular and influential methods for valuing investment opportunities.
What factors affect the time value of money?
Other factors can also influence the time value of money, or TVM. For example, inflation naturally increases over time, and that can lower the purchasing power of future dollars. In short: Money you can put to work now is usually worth more than the same amount down the line.