Calculating percent error can seem intimidating at first, but it’s actually quite simple once you break it down step-by-step. In this beginner’s guide, I’ll walk you through percent error calculations using easy-to-understand examples and a straightforward formula. Soon, you’ll be finding percent error like a pro!
What Exactly is Percent Error?
Let’s start with the basics – what is percent error anyway?
Percent error compares an experimental or measured value to the accepted or true value It gives you an idea of how far off your experiment or calculation is from the real result,
For example, let’s say you measured the width of a table as 268 inches But the actual width is 2.70 inches. The percent error would compare your measured value (2.68 in) to the true value (2.70 in) to see how close you were to the right width.
Percent error is especially useful in science experiments and measurement-based math problems. It lets you quantify how much your results deviate from the expected outcome. The lower the percent error, the more precise and accurate your experiment or calculation was.
Percent Error Formula – Simple as 1, 2, 3
The formula for calculating percent error is surprisingly easy. It involves just 3 quick steps:
Step 1: Find the Error
Subtract your experimental or measured value from the accepted or true value. This gives you the error amount.
For our table width example:
True value = 2.70 in
Measured value = 2.68 in
Error = True value – Measured value
= 2.70 in – 2.68 in
= 0.02 in
The error is 0.02 in.
Step 2: Divide Error by True Value
Take the error amount and divide it by the true or accepted value.
For our example:
Error = 0.02 in
True value = 2.70 in
0.02 in / 2.70 in = 0.0074074
This gives us a decimal representing the relative size of the error compared to the true value.
Step 3: Convert to Percentage
The last step is to convert the decimal from Step 2 into a percentage. You do this by multiplying it by 100%.
For our example:
0.0074074 x 100% = 0.74074%
Therefore, the percent error is 0.74074% or about 0.74%.
And that’s it! By following these 3 simple steps, you can easily calculate the percent error for any measured values compared to true, expected, or accepted values.
Let’s Try Some Examples
Now that you know the basic formula, let’s practice calculating percent error with a few different examples.
Example 1: Measuring the Length of a Pencil
Let’s say you need to measure the length of a pencil for a math assignment. You measure it 3 times and get these values:
- Trial 1: 6.7 inches
- Trial 2: 6.5 inches
- Trial 3: 6.6 inches
The actual length of the pencil is 6.9 inches. What is the percent error of each of your measurements compared to the true length?
For Trial 1:
True value = 6.9 in
Measured value = 6.7 in
Error = True value – Measured value = 6.9 – 6.7 = 0.2 in
Decimal = Error/True value = 0.2/6.9 = 0.02899
Percent error = Decimal x 100% = 0.02899 x 100% = 2.899%
Therefore, the percent error for Trial 1 is 2.899%
Follow the same process for Trials 2 and 3:
Trial 2:
Error = 0.4 in
Decimal = 0.4/6.9 = 0.05797
Percent error = 5.797%
Trial 3:
Error = 0.3 in
Decimal = 0.3/6.9 = 0.04348
Percent error = 4.348%
So Trial 2 had the highest percent error at 5.797% compared to the true length.
Example 2: Accuracy of a Basketball Shot
Let’s look at an example from sports. Say you take 10 shots from the free throw line in basketball. You make 7 of them. The true free throw percentage for experienced players is 75%. What is your percent error?
Measured value = You made 7/10 shots = 70%
True value = 75% free throw average
Error = True value – Measured value = 75% – 70% = 5%
Decimal = Error/True value = 5/75 = 0.0667
Percent error = Decimal x 100% = 0.0667 x 100% = 6.67%
So if the expected free throw percentage is 75% and you shot 70%, your percent error is 6.67%. Not too shabby! With a bit more practice, you’ll be matching the experts in no time.
Example 3: Accuracy of a Physics Experiment
Here’s an example from a physics lab experiment. You drop a ball to measure the acceleration due to gravity (g). Based on your measurements, you calculate g as 9.7 m/s2. But the accepted value for g is 9.8 m/s2. What’s the percent error of your experiment?
Measured value = 9.7 m/s2
True value = 9.8 m/s2
Error = True value – Measured value = 9.8 – 9.7 = 0.1 m/s2
Decimal = Error/True value = 0.1/9.8 = 0.01020
Percent error = Decimal x 100% = 0.01020 x 100% = 1.020%
So your measurement of g has a percent error of 1.020% compared to the true value. Your experiment was very accurate!
Tips for Minimizing Percent Error
Now that you know how to calculate percent error, here are some quick tips for minimizing it in your experiments and measurements:
-
Take multiple measurements and average the results to reduce random errors.
-
Use precise instruments correctly calibrated to provide accurate readings.
-
Refine your experiment and calculation methods. Simplify procedures and reduce uncertainties.
-
Report percent error properly using the right number of significant figures. Don’t round too soon.
-
Double check your measurements and calculations to avoid introducing human mistakes.
With careful technique and multiple trials, you can get your percent errors down to very low, acceptable levels.
Common Uses and Importance of Percent Error
Percent error is commonly used:
-
In physics, chemistry, and biology labs to assess accuracy and precision of experiments
-
In mathematical modeling and analysis to compare expected vs experimental results
-
In manufacturing and quality control to monitor deviations in product dimensions and specifications
-
In polling and surveys to determine the margin of error in results
-
In sports performance analytics to evaluate differences between predicted and actual outcomes
-
In diagnostics and medical testing to evaluate the reliability of screening and diagnostic methods
Understanding percent error provides valuable insight into the variability and reproducibility of scientific processes, experiments, manufacturing, testing, polling, and mathematical predictions. It provides a numerical assessment of accuracy and precision.
Lower percent errors indicate higher quality, more reliable processes and results. By calculating and minimizing percent error, we can have greater confidence in experimental findings, poll results, manufacturing standards, medical tests, and mathematical models.
The Takeaway – You’re Now a Percent Error Pro!
And with that, you should have a solid understanding of percent error calculations and applications! We walked through:
-
The definition and purpose of percent error
-
The easy 3-step formula for finding percent error
-
Step-by-step examples of calculating percent error
-
Tips for minimizing percent error in experiments
-
Common uses and importance across science, math, manufacturing, diagnostics, and more
Percent error is an extremely useful tool for quantifying the accuracy and variability of measured values compared to true, expected, or accepted values. By mastering these straightforward calculations, you can now confidently find, analyze, and minimize percent error in your work!
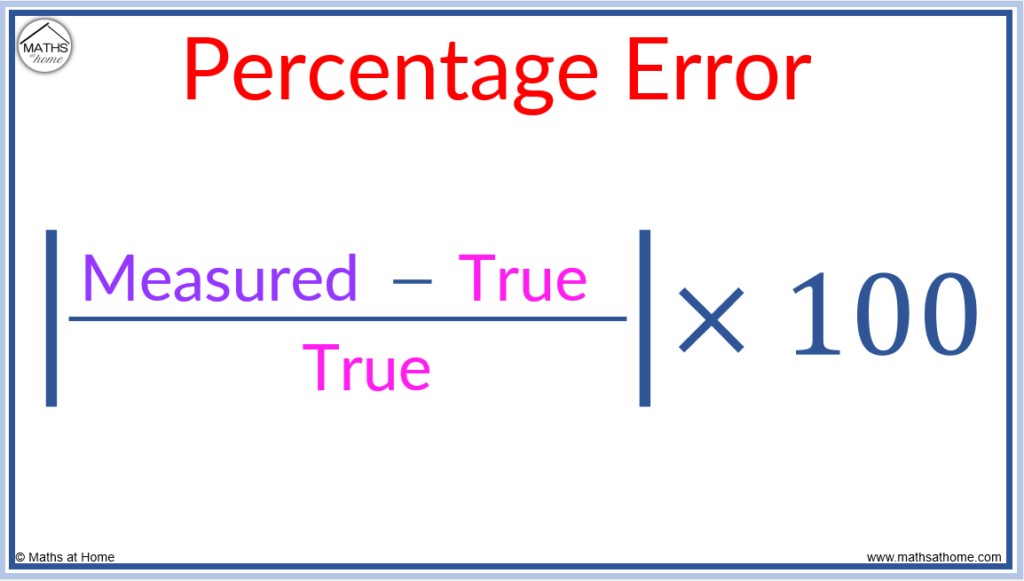
Formula for Percent Error
The formula to calculate Percent Error is:
| Percentage Error = [(Approximate Value – Exact Value) / Exact Value] × 100 |
Example 1: A scale measures wrongly a value as 8 cm due to some marginal errors. Calculate the percentage error if the actual measurement of the value is 12 cm.
Solution:
Given, Approximate value = 8 cm Exact value = 12 cm
Percentage Error = (Approximate Value – Exact Value / Exact Value × 100)
Percentage Error = (8 – 12)/12 × 100