We are familiar with the terms Domain of a Function and Range of a Function. But what does it mean? Before diving deeper into the topic, let us understand what a function is? Then, get into the detailed explanation of the domain, range and codomain of a function, along with solved examples.
Functions are one of the fundamental concepts in mathematics which have got numerous applications in the real world. Be it the mega skyscrapers or super-fast cars, their modelling requires methodical application of functions. Almost all real-world problems are formulated, interpreted, and solved using functions.
An understanding of relations is required to learn functions, Knowledge of Cartesian products is also required to learn about relations in maths. A Cartesian product of two sets A and B is the collection of all the ordered pairs (a, b) such that a ∈ A and b ∈ B.
A relation is a subset of a Cartesian product. Thus, a relation is a rule that “relates” an element from one set to another. A function is a special kind of relation. Let’s consider a relation F from A set A to B
Definition: A relation F is said to be a function if each element in set A is associated with exactly one element in set B.
This is because elements of set A are associated with more than one element of set B.
Suppose we define a relation F from set A to B such that it associates the countries with the year in which they won the world cup for the first time. Thus, every element in set A will be exactly associated with only one element in set B.
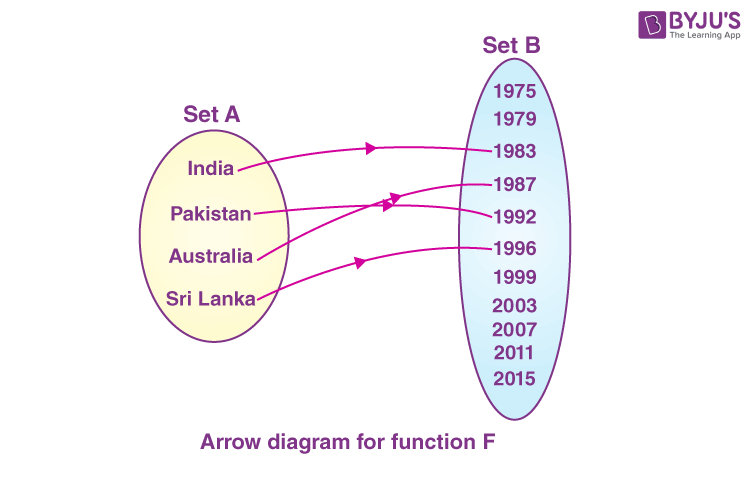Remember that in the case of a relation, the domain might not be the same as the left set in the arrow diagram. This is because the set may contain any element which doesn’t have an in the right set. But in the case of functions, the domain will always be equal to the first set. Range and Codomain of a function are defined in the same way as they are defined for relations.
The domain and range of a function can be identified based on the possibility of the given function to be defined in the real set. Let’s have a look at Domain and Range that is given in detail here.
The set of all possible values which qualify as inputs to a function is known as the domain of the function, or it can also be defined as the entire set of values possible for independent variables. The domain can be found in – the denominator of the fraction is not equal to zero and the digit under the square root bracket is positive. (In the case of a function with fraction values).
For e.g. the domain of the function F is set A i.e. {India, Pakistan, Australia, Sri Lanka}.
Finding the range of a function is an important concept in algebra and calculus. The range tells you the set of possible output values for a function. Knowing how to find the range allows you to understand the behavior of a function and how it transforms input values into output values. In this beginner’s guide, I will explain step-by-step how to find the range of any function in simple terms.
What is the Range of a Function?
The range of a function is the set of all possible output values the function can produce For any input value you put into the function, the output will be some value within the range
For example, consider the simple function f(x) = x + 5. For this function, if you input 2, the output is 2 + 5 = 7. If you input 10, the output is 10 + 5 = 15. The range of this function is all real numbers, since you can input any real number and get a real number as output.
The range is different from the domain of a function. The domain is the set of all allowed input values. The range is the set of all possible output values. To find the range, you examine the outputs while to find the domain, you look at the allowed inputs.
Step 1: Label the Function
The first step in finding the range is to clearly identify the function. Write the function with the output labeled explicitly as a variable like y.
For example if the function is f(x) = x2 – 3, rewrite this as y = x2 – 3
Giving the output a label like y makes it easier to see the connection between x and y and identify the range as values of y.
Step 2: Express x in Terms of y
After labeling the output as y express x as a function of y instead of the other way around.
For example, if the function is y = x2 – 3, rewrite this as:
x = ±√(y + 3)
This expresses x in terms of y. Now we can see that for any output value y, the input x must satisfy this equation.
Step 3: Find All Values of y Where f(y) is Defined
The third step is to find all possible values of y for which f(y) is defined.
For the function y = x2 – 3, f(y) is defined for all real numbers. There are no values of y that would make this function undefined.
However, for a function like y = √x, f(y) would be undefined for y < 0 because the square root of a negative number is imaginary.
So you must determine the set of all y-values where the function is defined. This is the set of all valid outputs.
Step 4: Eliminate Any Values That Make the Function Undefined
Based on step 3, eliminate any values of y that would make the function undefined.
For the square root function, we would eliminate all y < 0 because those y-values would require calculating the square root of a negative number which is undefined.
This narrows down the set of possible y-values to those that keep the function defined.
Step 5: Write the Range
Finally, write the range as the set of possible y-values based on the constraints identified from steps 3 and 4.
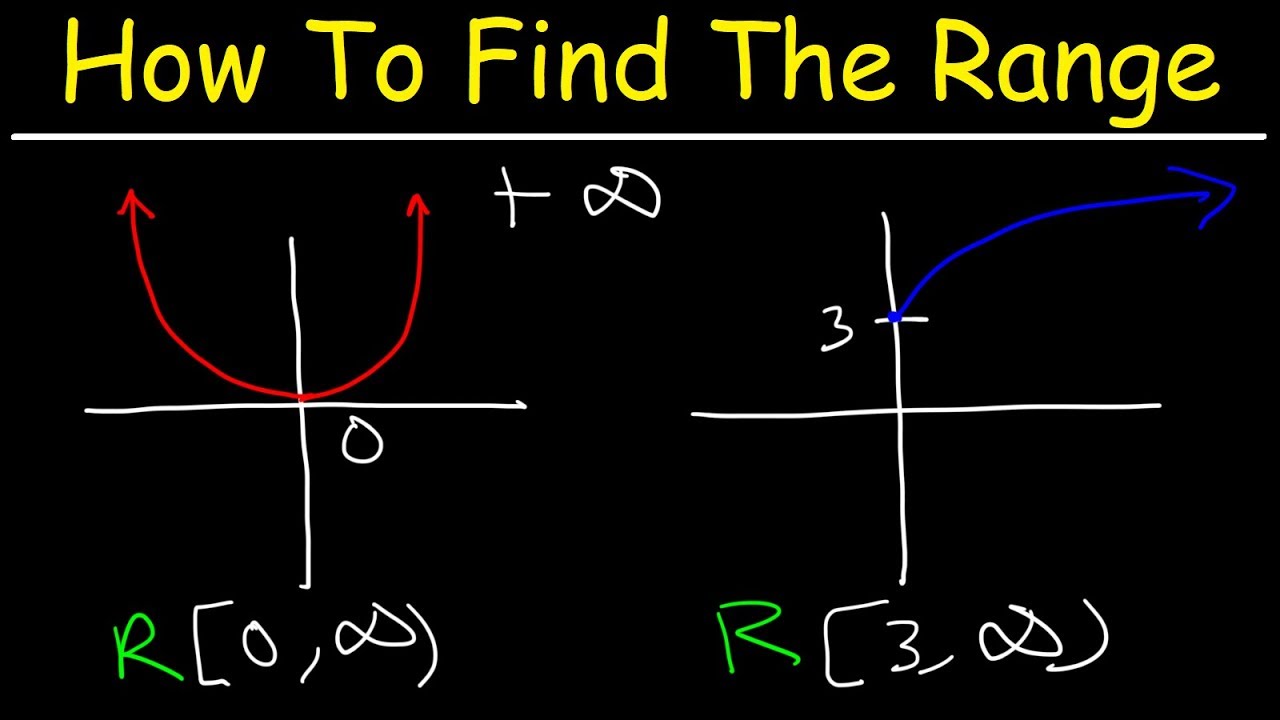
For a simple function like y = x2 – 3, the range is all real numbers or (-∞, ∞). For a function like y = √x, the range is [0, ∞) or all non-negative real numbers.
And that’s it! By following these 5 steps, you can find the range of any function. The key is labeling the output, expressing x in terms of y, finding possible y-values that maintain definition, eliminating any undefined cases, and writing the range.
Examples Finding the Range
Let’s look at a few examples to demonstrate how to follow these steps to find the range:
Example 1:
f(x) = x2 + 1
Step 1: Label output as y, so the function is
y = x2 + 1
Step 2: Express x in terms of y:
x = ±√(y – 1)
Step 3: f(y) is defined for all real y
Step 4: No values make f(y) undefined
Step 5: Range is (-∞, ∞)
Example 2:
f(x) = √x – 2
Step 1: y = √x – 2
Step 2: x = (y + 2)2
Step 3: f(y) defined for y ≥ -2
Step 4: Eliminate y < -2
Step 5: Range is [-2, ∞)
I hope these examples help illustrate how to systematically follow the steps to finding the range of any function. With some practice, this process becomes second nature.
When to Use Other Strategies
For more complex functions, you may need to use other strategies besides this step-by-step process:
-
For piecewise defined functions, evaluate the range separately for each piece.
-
For trigonometric functions like sin(x) and cos(x), the range is bounded based on their period.
-
For functions with absolute value like |x|, sketch the graph to visualize the range.
The five steps work well for simpler polynomial, radical, rational, and exponential functions. But sometimes creativity is needed for more involved functions.
Finding the range of a function is a fundamental skill in mathematics. Understanding the valid set of outputs allows you to properly interpret and graph functions. By methodically following these steps, you can determine the range of most basic functions:
-
Label the output as y
-
Express x in terms of y
-
Find possible values of y where f(y) is defined
-
Eliminate values that make f(y) undefined
-
Write the range
Range of a Function
How to Find the Range of a Function
Consider a function y = f(x).
- The spread of all the y values from minimum to maximum is the range of the function.
- In the given expression of y, substitute all the values of x to check whether it is positive, negative or equal to other values.
- Find the minimum and maximum values for y.
- Then draw a graph for the same.
In relations and functions, the codomain is the set of all possible outcomes of the given relation or function. Sometimes, the codomain is also equal to the range of the function. However, the range is the subset of the codomain.
An interesting point about the range and codomain is that “it is possible to restrict the range (i.e. the output of a function) by redefining the codomain of that function”. For example, the codomain of f(x) must be the set of all positive integers or negative real numbers and so on. Here, the output of the function must be a positive integer and the domain will also be restricted accordingly in this case.
Till now, we have represented functions with upper case letters but they are generally represented by lower case letters. If f is a function from set A to B and (a,b) ∈ f, then f(a) = b. b is called the of a under f and a is called the pre of b under f.
Summary:
- The domain is defined as the entire set of values possible for independent variables.
- The Range is found after substituting the possible x- values to find the y-values.
Example 1:
Find the domain and range of a function f(x) = 3×2 – 5.
Solution:
Given function:
f(x) = 3×2 – 5
We know that the domain of a function is the set of input values for f, in which the function is real and defined.
The given function has no undefined values of x.
Thus, for the given function, the domain is the set of all real numbers.
Domain = [-∞, ∞]
Also, the range of a function comprises the set of values of a dependent variable for which the given function is defined.
Ley y = 3×2 – 5
3×2 = y + 5
x2 = (y + 5)/3
x = √[(y + 5)/3]
Square root function will be defined for non-negative values.
So, √[(y + 5)/3] ≥ 0
This is possible when y is greater than y ≥ -5.
Hence, the range of f(x) is [-5, ∞).
Example 2:
Find the domain and range of a function f(x) = (2x – 1)/(x + 4).
Solution:
Given function is:
f(x) = (2x – 1)/(x + 4)
We know that the domain of a function is the set of input values for f, in which the function is real and defined.
The given function is not defined when x + 4 = 0, i.e. x = -4
So, the domain of given function is the set of all real number except -4.
i.e. Domain = (-∞, -4) U (-4, ∞)
Also, the range of a function comprises the set of values of a dependent variable for which the given function is defined.
Let y = (2x – 1)/(x + 4)
xy + 4y = 2x – 1
2x – xy = 4y + 1
x(2 – y) = 4y + 1
x = (4y + 1)/(2 – y)
This is defined only when y is not equal to 2.
Hence, the range of the given function is (-∞, 2) U (2, ∞).
- Find the domain and range of the function f(x) = 1/x.
- Write the domain set for this function f(x) = 1/(x3 − 3×2 − 6x − 8).
- What is the domain, codomain and range of the function f(x) = 4/√(x – 1).
